Steady flow All the fluid particles that pass any given point, follow the same path at same speed. In steady flow streamlines never cross each other.
Laminar flow In laminar flow, the velocities of all the particles on any given streamline are equal.
Turbulent flow Above a certain critical speed,fluid become turbulent .It is an irregular flow ,we can’t predict the motion.
Incompressible fluid
In incompressible fluid change in pressure produces no change in density of the fluid. Liquids can be considered incompressible and gases can be considered for small pressure differences.
Viscous force
When two layers of liquid are moving with different velocities they experience tangental forces which tend to retard the faster layer and accelerate the slower layer.These forces are called viscous forces.
Ideal fluid flow means a fluid incompressible,non viscous at streamline flow.
If a fluid is undergoing streamline flow then the mass of fluid which enters one end of a tube of flow must be equal to the mass that leaves at the other end during same time.
10 m3/h of water flows through a pipe with 100 mm inside diameter. The pipe is reduced to an inside dimension of 80 mm.
Using equation (2) the velocity in the 100 mm pipe can be calculated
(10 m3/h) (1 / 3600 h/s) = v100 (3.14 (0.1 m)2 / 4)
or
v100 = (10 m3/h) (1 / 3600 h/s) / (3.14 (0.1 m)2 / 4)
= 0.35 m/s
Using equation (2) the velocity in the 80 mm pipe can be calculated
(10 m3/h) (1 / 3600 h/s) = v80 (3.14 (0.08 m)2 / 4)
or
v80 = (10 m3/h) (1 / 3600 h/s) / (3.14 (0.08 m)2 / 4)
= 0.55 m/s
The kinetic energy of a moving fluid is more useful in applications like the Bernoulli equation when it is expressed as kinetic energy per unit volume

The potential energy of a moving fluid is more useful in applications like the Bernoulli equation when is expressed as potential energy per unit volume

Pressure in a fluid may be considered to be a measure of energy per unit volume or energy density. For a force exerted on a fluid, this can be seen from the definition of pressure:
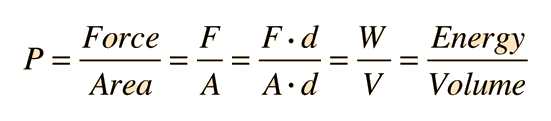
The Bernoulli Equation can be considered to be a statement of the conservation of energy principle appropriate for flowing fluids.
Applications
One of the most common everyday applications of Bernoulli’s principle is in air flight. The main way that Bernoulli’s principle works in air flight has to do with the architecture of the wings of the plane. In an airplane wing, the top of the wing is somewhat curved, while the bottom of the wing is totally flat. While in the sky, air travels across both the top and the bottom concurrently. Because both the top part and the bottom part of the plane are designed differently, this allows for the air on the bottom to move slower, which creates more pressure on the bottom, and allows for the air on the top to move faster, which creates less pressure. This is what creates lift, which allows planes to fly. An airplane is also acted upon by a pull of gravity in which opposes the lift, drag and thrust. Thrust is the force that enables the airplane to move forward while drag is air resistance that opposes the thrust force.
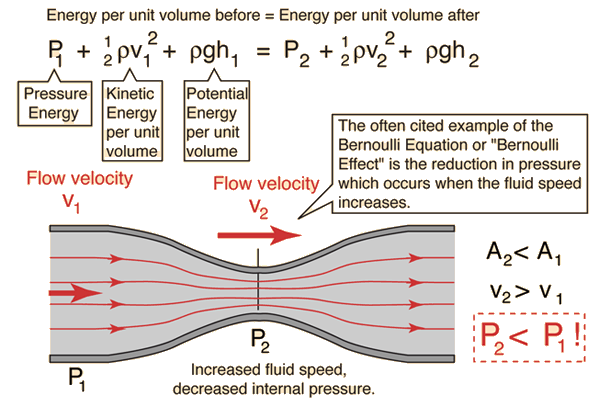
The Venturi effect is the reduction in fluid pressure that results when a fluid flows through a constricted section of a pipe.
In fluid dynamics, a fluid’s velocity must increase as it passes through a constriction in accord with the principle of mass continuity, while its static pressure must decrease in accord with the principle of conservation of mechanical energy. Thus any gain in kinetic energy a fluid may accrue due to its increased velocity through a constriction is balanced by a drop in pressure.
By measuring the change in pressure, the flow rate can be determined, as in various flow measurement devices such as venturi meters, venturi nozzles and orifice plates.
Using Bernoulli’s equation in the special case of steady, incompressible, non viscous flows along a streamline, the theoretical pressure drop at the constriction is given by:

where 


